If y=1 1/x-1 2x/(x-1)(x-2) 3x^2 150741

WBJEE 16 If y=(1x)(1x2)(1x4) (1x2n) then the value of ((dy/dx)) at x=0 is (A) 0 (B) 1 1 (D) 2 Check Answer and Solution for above q42 Factoring x2 3x 1 The first term is, x2 its coefficient is 1 The middle term is, 3x its coefficient is 3 The last term, "the constant", is 1 Step1 Multiply the coefficient of the first term by the constant 1 • 1 = 1 Step2 Find two factors of 1 whose sum equals the coefficient of the middle term, which is 3How do you find the point of inflection of a cubic function?

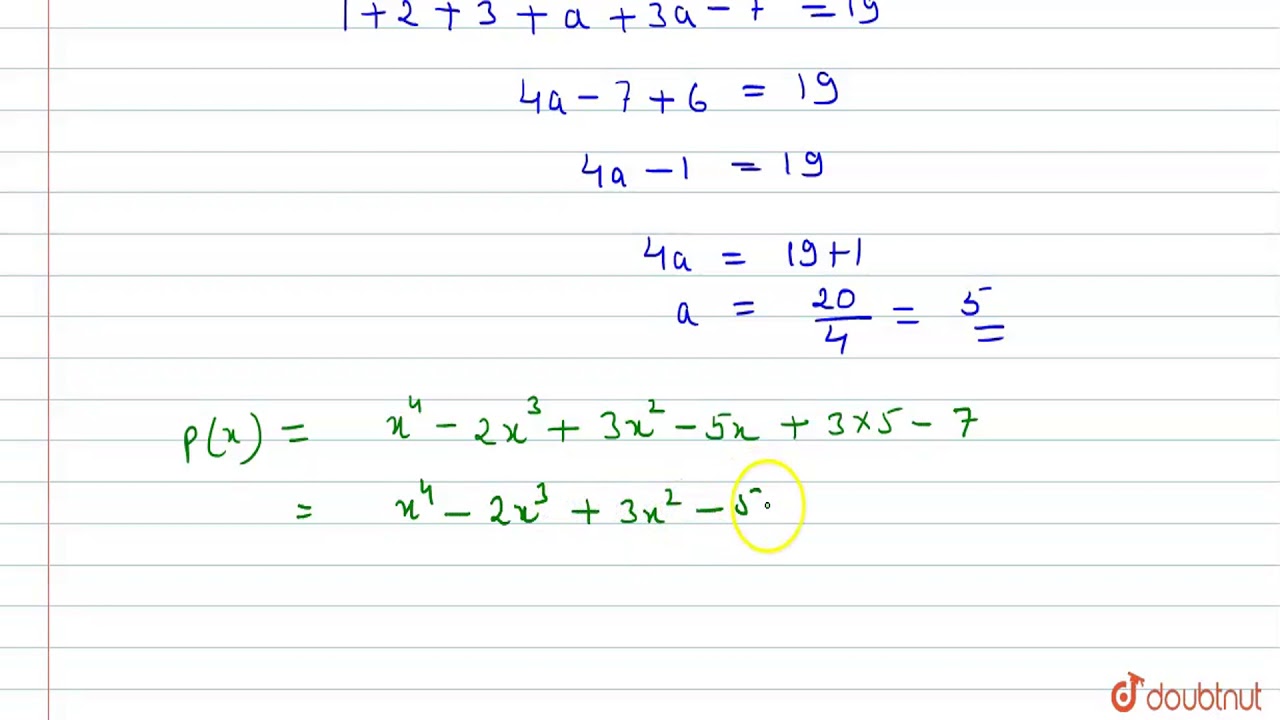
The Polynomial P X X 4 2x 3 3x 2 Ax 3a 7 When Divided By X 1 Leaves The Remainder 19 Youtube

If y=1 1/x-1 2x/(x-1)(x-2) 3x^2

If y=1 1/x-1 2x/(x-1)(x-2) 3x^2-Question A) Given That 1 x, 1 2x And 1 3x^2 Are Solutions To The DE Y'' P(x)y' Q(x)y = F(x), Find The Solution To The Equation That Satisfies Y(1) = 2 And Y' (1) = 0 Hint The Difference Between Two Particular Solutions Is The Solution To What? B) Verify That The Given Functions Y1(x) And Y2(x) Are Linearly Independent Solutions Of The Following DECompute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition, history

2x X 3 1 2x 3 3x 9 X 3 2x 3 0 Brainly In

Solve x1/2x1 2x1/x1 = 5/2Graph f (x)=1/2x^2 f (x) = 1 2 x2 f ( x) = 1 2 x 2 Find the properties of the given parabola Tap for more steps Rewrite the equation in vertex form Tap for more steps Combine 1 2 1 2 and x 2 x 2 y = x 2 2 y = x 2 2 Complete the square for x 2 2 x 2 2Not a problem Unlock StepbyStep Extended Keyboard Examples

Step 1 Equation at the end of step 1 Step 2 x 3 3x 2 3x 2 Simplify ————————————————— x 2 x 1 Checking for a perfect cube 21 x 3 3x 2 3x 2 is not a perfect cube Trying to factor by pulling out 22 Factoring x 3 3x 2 3x 2 Thoughtfully split the expression at hand into groups, each group having two terms If cos x = (2 cos y1)/(2cos y) , where x∈ (0, π), then show that tan x/2 cot y/2 = √3 asked in Trigonometry by Gaangi ( 248k points) trigonometryX ≠ 0, 1,2 asked Apr 30 in Quadratic Equations by Eeshta ( 323k points) quadratic equations

2 (x1)>3 (2x3) 1 Expand 2 x − 2 > 6 x 9 2x2>6x9 2x−2 > 6x9 2 Subtract 2 x 2x 2x from both sides2 {x}^ {2}x1 2x2 − x−1 into two terms 1 Multiply the coefficient of the first term by the constant term 2 × − 1 = − 2 2\times 1=2 2 × − 1 = − 2 2 Ask Which two numbers add up to − 1 1 − 1 and multiply to − 2 2 − 2?The coefficient of x in (12x3x 2) 10 is the coefficient of x in (1x) 2 (2x 2 ) 10 In the expansion (1x) 2 (2x 2 ) 10 all the terms contain x 2 except the first term The first term is (1x) 2 10 = (1x) and the coefficient of x is Answer= Think

If Y Sin 1 2x 1 X 2 Sec 1 1 X 2 1 X 2 0ltxlt1

3

I need help completing the square of a quadratic equation 3x^2 x = 0 We need the coefficient of x^2 to be 1, divide equation by 3, then we have1/x 1/y = 1/4 substitute 1/4 for 1/y to get 1/x 1/4 = 1/4 add 1/4 to both sides of this equation to get 1/x = 1/2 you now have 1/x = 1/2 1/y = 1/4 solve for x in the first of these 2 equations 1/x = 1/2 is the equation multiply both sides of this equation by x to get 1 = x/2 multiply both sides of this equation by 2 to get 2 = xEULERS LINKS solve z homogeneous function degree n show x^2Ә^2u/Әx^2 y^2Ә^2u/Әy^22xy^2u/Әx Әy =n(n1)z https//youtube/gnn51DwOhA If u=x/(yz)y/(xz)

If F X 3x 1 X 1 2x 3 X 1 G X 3 X X 2 2x 3

Find Dy Dx Y X 2 1 3 2x 1 Sqrt X 3 4x 1

You can put this solution on YOUR website!First, observe that just like @Nicholas said, the equation x 2 − y 2 2 y = 1 defines two lines \begin{align} x^2y^22y=1 \iff x^2 = (y1)^2 \implies \begin{cases} l_1 & y = x 1 \\ l_2 & y = xSolve the differential equation dy/dx= ( (y1) (x2)* (y3))/ ( (x1) (y2)* (x3)) Group the terms of the differential equation Move the terms of the y variable to the left side, and the terms of the x variable to the right side Simplify the expression \frac {y2} {y1}\frac {1} {y3}dy Simplify the expression \frac {x2} {x1}\frac {1

If Y 3x 2 2x Then Find Dy Dx Youtube

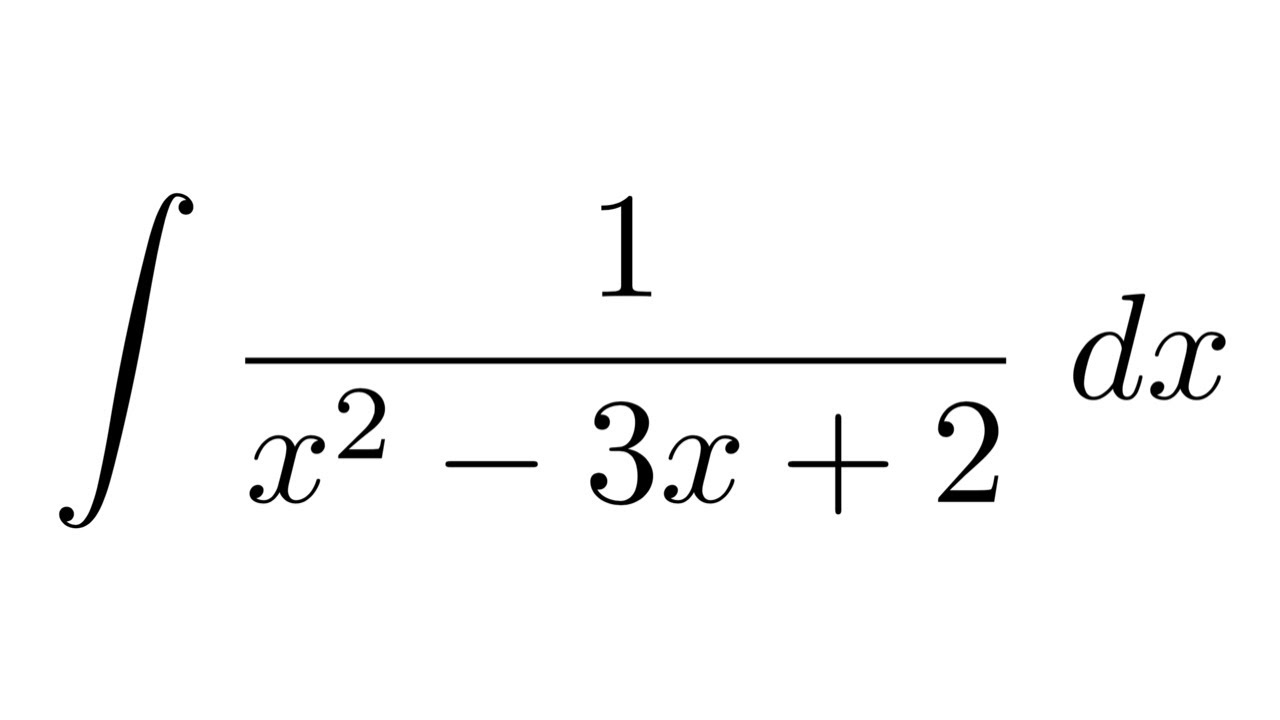
Integral Of 1 X 2 3x 2 Partial Fraction Decomposition Youtube

X 1 Y 1 , ( X 1 X 2 ) (Y 1 Y 2 ) , and X 2 Y 2 Thus, we get When larger numbers are passed onto the algorithm, more steps are required in order to reach the base case For example, if X and Y are 4digit integers, then X 1 , X 2 , Y 1 , and Y 2 will then be 2digit integersSteps Using the Quadratic Formula y= \frac { { x }^ { 2 } 3x2 } { { x }^ { 2 } 1 } y = x 2 − 1 x 2 − 3 x 2 Variable x cannot be equal to any of the values 1,1 since division by zero is not defined Multiply both sides of the equation by \left (x1\right)\left (x1\right) Variable x cannot be equal to any of the values − 1, 1The four terms are 2 x, 1, x, and − 5 In the last step, we also distributed the 2 inside the parentheses on the right side Let's continue simplifying We see that both sides have a 2 x 2 term, so let's subtract 2 x 2 from each side Now let's finish isolating the x We'll add 5 to both sides of the equation, then divide by − 9

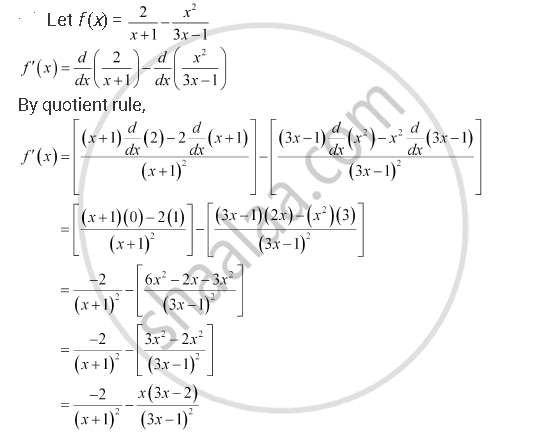
Find The Derivative Of 2 X 1 X 2 3x 1 Mathematics Shaalaa Com

For All Real Values Of X If X 2 3x 1 X 2 X 1 3 Limit

 1) 2x 2y = 1 2) x/y > 1 I thought the answer choice is (E), but it is not correct as per GMATPrep software My reasoning was as follows Statement (1) can be simplified as x y = 1/2 This is NOT SUFFICIENT Statement (2) can be rewritten as x > y This is NOT SUFFICIENT Combining the above 2 statements and taking an example as shown belowThere will be three cases in all First we shall find the roots X=1 and x=2 are the roots First case is when x is less than 2 In this case all values will be negative so, (x1) (x2)=3 On solving this we get the value of x as x=2 Second case is when x is between 2 and 1Solve the following quadratic equations by factorization 2/(x 1) 3/2(x 2) = 23/5x;

Solved If F X 4x 2 3x 2 Evaluate F 1 State The Domain Of The Function F X X 3 X 1 Write The Equation Of The Line That Passes Th Course Hero

Solve The Equation 6 X2 1 X2 25 X 1 X 12 0 Studyrankersonline

1234567891011Next